volume of revolution about a slanted line

Tag Archives: volume of revolution about a slanted line

January 1, 2025 · 9:40 am

Volume of Rotation About a Slanting Line

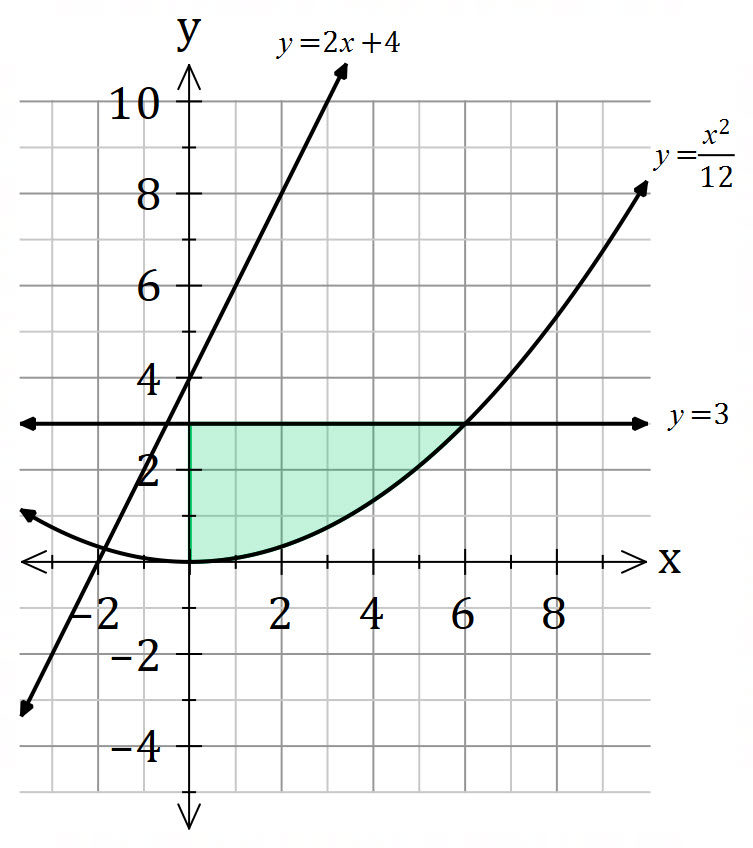
Given the area in the first quadrant bounded by $x^2=12y$ , the line $y=3$ and the $y-$ axis. What is the volume generated when this area is rotated about the line $2x-y+4=0$ ?

Rotate the green region about the line $y=2x+4$

Rotate the green region about the line $y=2x+4$

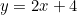
We can split the solid into shells.

$\begin{equation*}V=2\pi r dx dy\end{equation}$

Where $r$ is the distance from each $(x,y)$ point in the region to the line $2x-y+4=0$ , $dx$ is the width, and $dy$ is the height.

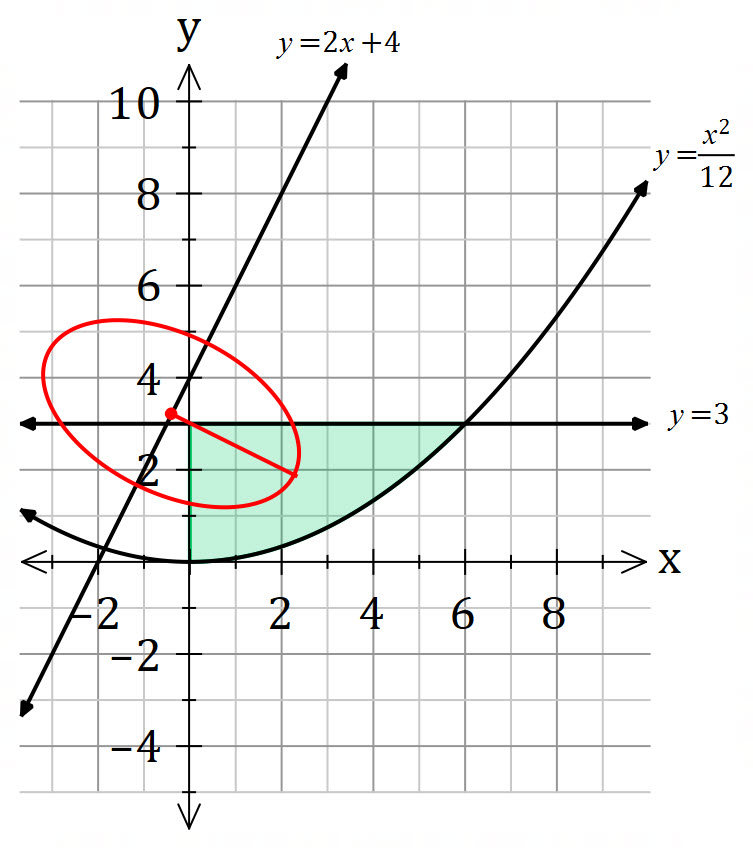
The distance between a point and a line is

$\begin{equation*} d=\frac{Ax+By+C=0}{\sqrt{A^2+B^2}}\end{equation}$

Hence, $r=\frac{2x-y+4}{\sqrt{5}}$

$\begin{equation*}V=2\pi\int \int \frac{2x-y+4}{\sqrt{5}} dx dy\end{equation}$

Now we just need to work out the bounds.

$0\le y \le 3$ and $0\le x\le \sqrt{12y}$

$\begin{equation*}V=\frac{2\pi}{\sqrt{5}} \int_0^3 \int_0^{\sqrt{12y}} 2x-y+4 dx dy\end{equation}$

$\begin{equation*}V=\frac{2\pi}{\sqrt{5}}\int_0^3 x^2-yx+4x]_0^{\sqrt{12y}} dy \end{equation}$

$\begin{equation*}V=\frac{2\pi}{\sqrt{5}}\int_0^3 12y-\sqrt{12}y^{\frac{3}{2}+4\sqrt{12y} dy\end{equation}$

$\begin{equation*}V=\frac{2\pi}{\sqrt{5}}(6y^2-\frac{2\sqrt{12}}{5}y^{\frac{5}{2}}+\frac{8\sqrt{12}}{3}y^{\frac{3}{2}}]_0^3\end{equation}$

$\begin{equation*}V=\frac{2\pi}{\sqrt{5}}(54-\frac{2\sqrt{12}}{5}(9\sqrt{3})+\frac{8\sqrt{12}}{3}(3\sqrt{3}))\end{equation}$

$\begin{equation*}V=\frac{2\pi}{\sqrt{5}}(54-\frac{108}{5}+48)\end{equation}$

$\begin{equation*}V=\frac{804\pi}{\sqrt{5}}\end{equation}$

If we rationalise the denominator

$\begin{equation*}V=\frac{804\sqrt{5}\pi}{25}\end{equation}$

Tag Archives: volume of revolution about a slanted line

Volume of Rotation About a Slanting Line

Recent Posts

Categories

Archives