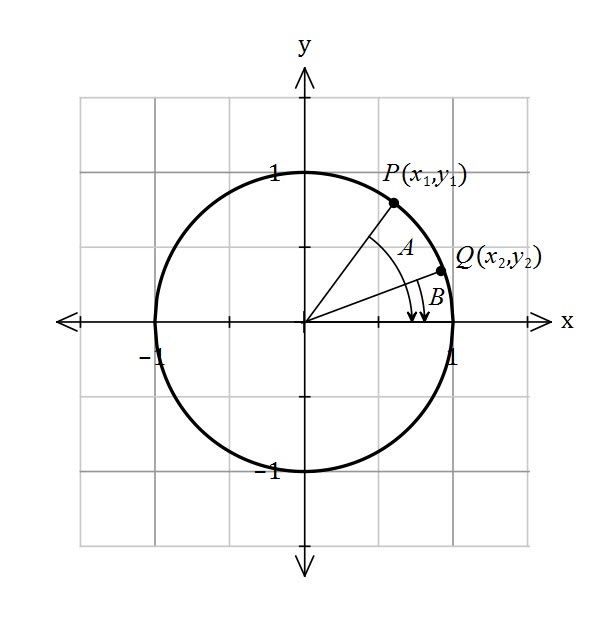
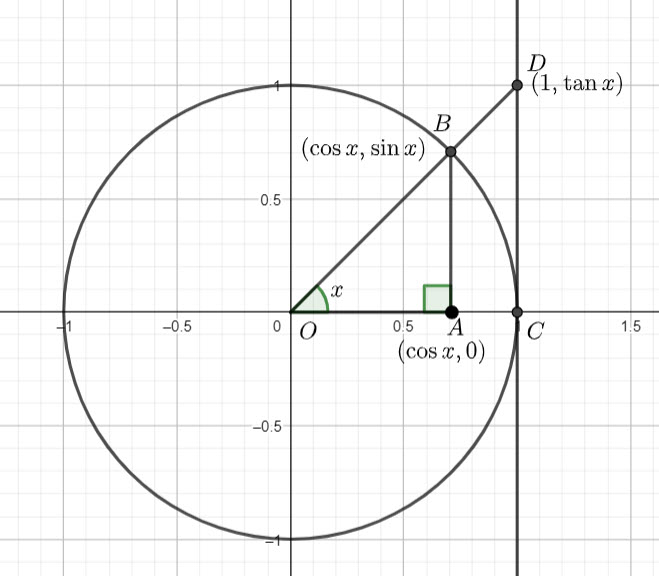
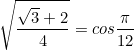Category Archives: Identities

November 26, 2025 · 10:57 am

Trigonometric Equation

Solve $cos(4\theta)+cos(2\theta)+cos(\theta)=0$ for $0\le \theta \le\pi$

Remember the identity

(1) $\begin{equation*}cos(A)+cos(B)=2cos(\frac{A+B}{2})cos(\frac{A-B}{2})\end{equation*}$

Hence

$\begin{equation*}cos(4\theta)+cos(2\theta)=2cos(3\theta)cos(\theta)\end{equation}$

Now I have

$\begin{equation*}2cos(3\theta)cos(\theta)+cos(\theta)=0\end{equation}$

$\begin{equation*}cos(\theta)(2cos(3\theta)+1)=0\end{equation}$

$cos(\theta)=0$ or $cos(3\theta)=\frac{-1}{2}$

$\theta=\frac{\pi}{2}$

$cos(3\theta)=-\frac{1}{2}$ for $0 \le \theta \le 3\pi$

$3\theta=\frac{2\pi}{3}, \frac{4\pi}{3}, \frac{8\pi}{3}$

$\theta=\frac{2\pi}{9}, \frac{4\pi}{9}, \frac{8\pi}{9}$

Hence $\theta =\frac{\pi}{2},\frac{2\pi}{9}, \frac{4\pi}{9}, \frac{8\pi}{9}$

Leave a Comment

Filed under Identities, Quadratic, Solving Equations, Solving Trig Equations, Trigonometry, Year 11 Specialist Mathematics

Tagged as solving trig equations, sum to product trig identity

October 9, 2025 · 6:37 am

Trigonometric Exact Values

Find exactly $sin(18^\circ)$

We must be able to find an arithmetic combination of the exact values we knew to find $18$ .

$\begin{equation*}90=5\times 18\end{equation}$

$\begin{equation*}90-3(18)=2(18)\end{equation}$

I re-arranged as above, so I could take advantage of $cos(90)=0$ and $sin(90)=1$

cos(2x)=cos^2(x)-sin^2(x)=1-2sin^2(x)=2cos^2(x)-1

$\begin{equation*}sin(90-3(18))=sin(2(18))\end{equation}$

$\begin{equation*}sin(90)cos(3(18))-sin(3(18))cos(90)=2sin(18)cos(18)\end{equation}$

$\begin{equation*}cos(3(18))=2sin(18)cos(18)\end{equation}$

$\begin{equation*}4cos^3(18)-3cos(18)=2sin(18)cos(18)\end{equation}$

$\begin{equation*}4cos^3(18)-3cos(18)-2sin(18)cos(18)=0\end{equation}$

$\begin{equation*}cos(18)(4cos^2(18)-3-2sin(18))=0\end{equation}$

Hence,

$\begin{equation*}4cos^2(18)-2sin(18)-3=0\end{equation}$

$\begin{equation*}4-4sin^2(18)-2sin(18)-3=0\end{equation}$

$\begin{equation*}-4sin^2(18)-2sin(18)+1=0\end{equation}$

Use the quadratic equation formula

$\begin{equation*}sin(18)=\frac{-b\pm \sqrt{b^2-4ac}}{2a}\end{equation}$

$\begin{equation*}sin(18)=\frac{2 \pm \sqrt{4-4(-4)(1)}}{-8}\end{equation}$

$\begin{equation*}sin(18)=\frac{2 \pm \sqrt{20}}{-8}\end{equation}$

$\begin{equation*}sin(18)=\frac{-2 \mp 2\sqrt{5}}{8}\end{equation}$

$\begin{equation*}sin(18)=\frac{-1 \mp \sqrt{5}}{4}\end{equation}$

As $sin(18)>0$ , $sin(18)=\frac{-1+\sqrt{5}}{4}$

Leave a Comment

Filed under Addition and Subtraction Identities, Algebra, Identities, Quadratic, Quadratics, Solving, Solving Equations, Solving Trig Equations, Trigonometry, Year 11 Specialist Mathematics

Tagged as exact values, sin(18), trig exact values, trigonometric identities

August 25, 2025 · 3:29 am

Matrices -Linear Transformations (Rotation)

We are going to find a matrix to rotate a point about the origin a number of degrees (or radians).

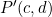
$P$ and $P'$ are equidistant from the origin. I.e. $\sqrt{c^2+d^2}=\sqrt{a^2+b^2}$

Remember, anti-clockwise angles are positive.

$\begin{equation*}cos(\theta+\alpha)=\frac{c}{\sqrt{c^2+d^2}}\end{equation}$

$\begin{equation*}c=\sqrt{a^2+b^2}cos(\theta+\alpha)\end{equation}$

Use the cosine addition identity.

$\begin{equation*}c=\sqrt{a^2+b^2}(cos(\theta)cos(\alpha)-sin(\theta)sin(\alpha))\end{equation}$

$\begin{equation*}c=\sqrt{a^2+b^2}(cos(\theta)\frac{a}{\sqrt{a^2+b^2}}-sin(\theta)\frac{b}{\sqrt{a^2+b^2}})\end{equation}$

(1) $\begin{equation*}c=acos(\theta)-bsin(\theta)\end{equation*}$

We will do the same for $d$

$\begin{equation*}sin(\theta+\alpha)=\frac{d}{\sqrt{a^2+b^2}}\end{equation}$

$\begin{equation*}d=\sqrt{a^2+b^2}sin(\theta+\alpha)\end{equation}$

Use the sine addition identity.

$\begin{equation*}d=\sqrt{a^2+b^2}(sin(\theta)cos(\alpha)+cos(\theta)sin(\alpha)\end{equation}$

$\begin{equation*}d=\sqrt{a^2+b^2}(sin(\theta)\frac{a}{\sqrt{a^2+b^2}}+cos(\theta)\frac{b}{\sqrt{a^2+b^2}})\end{equation}$

(2) $\begin{equation*}d=asin(\theta)+bcos(\theta)\end{equation*}$

Let $R$ be the rotation matrix, then

$\begin{equation*}R\begin{bmatrix}a\\b\end{bmatrix}=\begin{bmatrix}acos(\theta)-bsin(\theta)\\asin(\theta)+bcos(\theta)\end{bmatrix}\end{equation}$

Hence $R$ must be

(3) $\begin{equation*}R=\begin{bmatrix}cos(\theta)&-sin(\theta)\\sin(\theta)&cos(\theta)\end{bmatrix}\end{equation*}$

Example

Find the image of the line $y=x+1$ after it is rotated $60^\circ$ about the origin.

I am going to select two points on the line and transform them.

$\begin{equation*}\begin{bmatrix}cos(60)&-sin(60)\\sin(60)&cos(60)\end{bmatrix}\begin{bmatrix}0&4\\1&5\end{bmatrix}=\begin{bmatrix}x'_1&x'_2\\y'_1&y'_2\end{bmatrix}\end{equation}$

$\begin{equation*}\begin{bmatrix}\frac{1}{2}&-\frac{\sqrt{3}}{2}\\\frac{\sqrt{3}}{2}&\frac{1}{2}\end{bmatrix}\begin{bmatrix}0&4\\1&5\end{bmatrix}=\begin{bmatrix}x'_1&x'_2\\y'_1&y'_2\end{bmatrix}\end{equation}$

$\begin{equation*}\begin{bmatrix}-\frac{\sqrt{3}}{2}&\frac{4-5\sqrt{3}}{2}\\\frac{1}{2}&2\sqrt{3}+\frac{5}{2}\end{bmatrix}=\begin{bmatrix}x'_1&x'_2 \\y'_1&y'_2\end{bmatrix}\end{equation}$

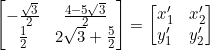
We can then find the equation of the line.

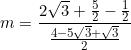
$\begin{equation*}m=\frac{2\sqrt{3}+\frac{5}{2}-\frac{1}{2}}{\frac{4-5\sqrt{3}+\sqrt{3}}{2}}\end{equation}$

$\begin{equation*}m=-2-\sqrt{3}\end{equation}$

$\begin{equation*}y-\frac{1}{2}=(-2-\sqrt{3})(x+\frac{\sqrt{3}}{2})\end{equation}$

$\begin{equation*}y=(-2-\sqrt{3})x-1-\sqrt{3}\end{equation}$

Leave a Comment

Filed under Addition and Subtraction Identities, Identities, Matrices, Transformations, Trigonometry, Year 11 Specialist Mathematics

Tagged as matrix transformations, rotation matrix

August 14, 2025 · 6:39 am

Trig Identities and Exact Values

My Year 11 Specialist Mathematics students are working on Trig identities. We came across this question

Without the use of a calculator, evaluate
(a) $cos20^\circ\times cos40^\circ\times cos80^\circ$

(b) $cos(\frac{\pi}{7})\times cos(\frac{2\pi}{7})\times cos(\frac{4\pi}{7})$

OT Lee Year 11 Specialist Mathematics textbook

I spent a bit of time thinking about the question. Can you use a product to sum identity twice? But I was always being left with an angle that doesn’t have a nice exact value.

I tried a few things, had a chat to Meta AI, and finally stumbled upon this method.

Remember

$\begin{equation*}sin(2x)=2sin(x)cos(x)\end{equation}$

Which can be rearranged to

$\begin{equation*}cos(x)=\frac{sin(2x)}{sin(x)}\end{equation}$

(a) $cos20^\circ\times cos40^\circ\times cos80^\circ=\frac{sin(40)}{2sin(20)}\frac{sin(80)}{2sin(40)}\frac{sin(160)}{2sin(80)}$

Which simplifies to

$\begin{equation*}\frac{sin(160)}{8sin(20)}\end{equation}$

Now $sin(160)=sin(20)$

Hence $cos20^\circ\times cos40^\circ\times cos80^\circ=\frac{1}{8}$

And we will do the same for part (b)

$cos(\frac{\pi}{7})\times cos(\frac{2\pi}{7})\times cos(\frac{4\pi}{7})=\frac{sin(\frac{2\pi}{7})}{2sin(\frac{\pi}{7})}\frac{sin(\frac{4\pi}{7})}{2sin(\frac{2\pi}{7})}\frac{sin(\frac{8\pi}{7})}{2sin(\frac{4\pi}{7})}$

Which simplifies to

$cos(\frac{\pi}{7})\times cos(\frac{2\pi}{7})\times cos(\frac{4\pi}{7})=\frac{sin(\frac{8\pi}{7})}{8sin(\frac{\pi}{7})}$

Now $sin(\frac{8\pi}{7})=-sin(\frac{\pi}{7})$

Hence $cos(\frac{\pi}{7})\times cos(\frac{2\pi}{7})\times cos(\frac{4\pi}{7})=\frac{-1}{8}$

And then I had to test them on my Classpad.

Leave a Comment

Filed under Classpad Skills, Identities, Simplifying fractions, Trigonometry, Year 11 Specialist Mathematics

Tagged as exact values, trig identities

August 10, 2025 · 4:54 am

Trigonometric Identities – Product to Sum

Let’s think about the sine and cosine addition and subtraction trig identities.

(1) $\begin{equation*}sin(A+B)=sinAcosB+cosAsinB\end{equation*}$

(2) $\begin{equation*}sin(A-B)=sinAcosB-cosAsinB\end{equation*}$

If we add equation $1$ and $2$ , we get

$\begin{equation*}sin(A+B)+sin(A-B)=2sinAcosB\end{equation}$

Hence, $sinAcosB=\frac{1}{2}(sin(A+B)+sin(A-B))$

If we subtract equation $2$ from equation $1$ , we get

$\begin{equation*}sin(A+B)-sin(A-B)=2cosAsinB\end{equation}$

Hence, $cosAsinB=\frac{1}{2}(sin(A+B)-sin(A-B)$

What about the cosine addition and subtraction idenities?

(3) $\begin{equation*}cos(A+B)=cosAcosB-sinAsinB\end{equation*}$

(4) $\begin{equation*}cos(A-B)=cosAcosB+sinAsinB\end{equation*}$

If we add equation $3$ and $4$ , we get

$\begin{equation*}cos(A+B)+cos(A-B)=2cosAcosB\end{equation}$

Hence, $cosAcosB=\frac{1}{2}(cos(A+B)+cos(A-B))$

If we subtract $3$ from $4$ , we get

$\begin{equation*}cos(A-B)-cos(A+B)=2sinAsinB\end{equation}$

Hence, $sinAsinB=\frac{1}{2}(cos(A-B)-cos(A+B))$

These are the product to sum identities.

$\begin{equation*}sinAcosB=\frac{1}{2}(sin(A+B)+sin(A-B))\end{equation}$

$\begin{equation*}cosAsinB=\frac{1}{2}(sin(A+B)-sin(A-B))\end{equation}$

$\begin{equation*}cosAcosB=\frac{1}{2}(cos(A+B)+cos(A-B))\end{equation}$

$\begin{equation*}sinAsinB=\frac{1}{2}(cos(A-B)-cos(A+B))\end{equation}$

Examples

(1) Solve $sin(5x)-sin(x)=0$ for $0\le x \le 2\pi$

Remember,

$\begin{equation*}cosAsinB=\frac{1}{2}(sin(A+B)-sin(A-B))\end{equation}$

$\begin{equation*}A+B=5\end{equation}$

$\begin{equation*}A-B=1\end{equation}$

Therefore, $A=3$ and $B=2$

$\begin{equation*}sin(5x)-sin(x)=2cos(3x)sin(2x)=0\end{equation}$

$\begin{equation*}cos(3x)=0\end{equation}$

$\begin{equation*}3x=\frac{\pi}{2},\frac{3\pi}{2}, \frac{5\pi}{2}, \frac{7\pi}{2}, \frac{\9\pi}{2}, \frac{11\pi}{2}\end{equation}$

$\begin{equation*}x=\frac{\pi}{6}, \frac{\pi}{2}, \frac{5\pi}{6}, \frac{7\pi}{6}, \frac{3\pi}{2}, \frac{11\pi}{6}\end{equation}$

$\begin{equation*}sin(2x)=0\end{equation}$

$\begin{equation*}2x=0, \pi, 2\pi, 3\pi, 4\pi\end{equation}$

$\begin{equation*}x=0, \frac{\pi}{2}, \pi, \frac{3\pi}{2}, 2\pi\end{equation}$

Hence $x=0, \frac{\pi}{6}. \frac{\pi}{2}, \frac{5\pi}{6}, \pi, \frac{7\pi}{6}, \frac{3\pi}{2}, \frac{11\pi}{6}, 2\pi$

(2)Solve $sin(7\theta)-sin(\theta)=sin(3\theta)$ for $0 \le \theta \le\2\pi$

$\begin{equation*}cosAsinB=\frac{1}{2}(sin(A+B)-sin(A-B))\end{equation}$

Therefore, $A+B=7$ and $A-B=1$

$A=4, B=3$

$\begin{equation*}2cos(4\theta)sin(3\theta)=sin(3\theta)\end{equation}$

$\begin{equation*}2cos(4\theta)sin(3\theta)-sin(3\theta)=0\end{equation}$

$\begin{equation*}sin(3\theta)(2cos(4\theta)-1)=0\end{equation}$

$sin(3\theta)=0$ and $cos(4\theta)=\frac{1}{2}$

$3\theta=0, \pi, 2\pi, 3\pi, 4\pi, 5\pi, 6\pi$

$\theta=0, \frac{\pi}{3}, \frac{2\pi}{3}, \frac{4\pi}{3}, \frac{5\pi}{3}. 2\pi$

$cos(4\theta)=\frac{1}{2}$

$4\theta=\frac{\pi}{3}, \frac{5\pi}{3}, \frac{7\pi}{3}, \frac{11\pi}{3}, \frac{13\pi}{3}, \frac{17\pi}{3}, \frac{19\pi}{3}, \frac{23\pi}{3}$

$\theta=\frac{\pi}{12}, \frac{5\pi}{12}, \frac{7\pi}{12}, \frac{11\pi}{12}, \frac{13\pi}{12}, \frac{17\pi}{12}, \frac{19\pi}{12}, \frac{23\pi}{12}$

Hence $\theta=0, \frac{\pi}{3}, \frac{5\pi}{12}, \frac{7\pi}{12}, \frac{11\pi}{12}, \frac{13\pi}{12}, \frac{17\pi}{12}, \frac{5\pi}{3}, \frac{23\pi}{12}, 2\pi$

Leave a Comment

Filed under Addition and Subtraction Identities, Identities, Product to Sum idenitites, Trigonometry

Tagged as product to sum trig identities, solving trig equations, trig identities

August 2, 2025 · 9:30 am

Trigonometric Exact Value

Using an appropriate double angle identity, find the exact value of
$cos(\frac{\pi}{12})$

The double angle identity for sine is

(1) $\begin{equation*}cos(2A)=cos^2A-sin^2A=2cos^2A-1=1-2sin^2A\end{equation*}$

That means $\frac{\pi}{12}$ is either $2A$ or $A$ .

It must be $A$ as $2\times\frac{\pi}{12}=\frac{\pi}{6}$ as there are exact values for $\frac{\pi}{6}$

Hence,

$\begin{equation*}cos{\frac{\pi}{6}}=2cos^2{\frac{\pi}{12}}-1\end{equation}$

$\begin{equation*}\frac{\sqrt{3}}{2}=2cos^2{\frac{\pi}{12}}-1\end{equation}$

$\begin{equation*}\frac{\sqrt{3}}{2}+1=2cos^2{\frac{\pi}{12}}\end{equation}$

$\begin{equation*}\frac{\frac{\sqrt{3}}{2}+1}{2}=cos^2{\frac{\pi}{12}}\end{equation}$

$\begin{equation*}\frac{\sqrt{3}+2}{4}=cos^2{\frac{\pi}{12}}\end{equation}$

$\begin{equation*}\sqrt{\frac{\sqrt{3}+2}{4}}=cos{\frac{\pi}{12}}\end{equation}$

As $\frac{\pi}{12}$ is in the first quadrant, we don’t need to consider the negative version.

$\begin{equation*}cos(\frac{\pi}{12})=\frac{\sqrt{3}+2}{2}\end{equation}$

Leave a Comment

Filed under Algebra, Identities, Trigonometry, Year 11 Mathematical Methods

Tagged as trig double angle identities, trig exact values

July 28, 2025 · 8:09 am

Trig Identities – Addition and Subtraction

Deriving the addition and subtraction trigonometric identities.

We will start with cosine, and use the result to derive the remaining identities.

Proving $cos(A-B)=cos(A)cos(B)+sin(A)(sin(B)$ .

$A$ and $B$ are represented in the unit circle below.

Remember $P(x_1,y_1)=(cos(A), sin(A))$ and $Q(x_2, y_2)=(cos(B), sin(B))$

Using the cosine rule and triangle $OPQ$ , find $PQ$

$\begin{equation*}(PQ)^2=1^2+1^2-2(1)(1)cos(A-B)\end{equation}$

$\begin{equation*}(PQ)^2=2-2cos(A-B)\end{equation}$

Using the distance between points, find $PQ$

$\begin{equation*}(PQ)^2=(x_1-x_2)^2+(y_1-y_2)^2\end{equation}$

$\begin{equation*}(PQ)^2=(cosA-cosB)^2+(sinA-sinB)^2\end{equation}$

$(PQ)^2=cos^2A-2cosAcosB+cos^2B+sin^2A-2sinAsinB+sin^2B$

Remember the Pythagorean identity

$\begin{equation*}cos^2\theta+sin^2\theta=1\end{equation}$

$\begin{equation*}(PQ)^2=2-2cosAcosB-2sinAsinB\end{equation}$

Hence

$\begin{equation*}2-2cos(A-B)=2-2cosAcosB-2sinAsinB\end{equation}$

(1) $\begin{equation*}cos(A-B)=cosAcosB+sinAsinB\end{equation*}$

We can then use this identity to find $cos(A+B)$ .

$\begin{equation*}cos(A+B)=cos(A-(-B))\end{equation}$

$\begin{equation*}cos(A-(-B))=cos(A)cos(-B)+sinAsin(-B)\end{equation}$

Remember $cos(-B)=cos(B)$ and $sin(-B)=-sin(B)$

$\begin{equation*}cos(A-(-B))=cosAcosB-sinAsinB\end{equation}$

(2) $\begin{equation*}cos(A+B)=cosAcosB-sinAsinB\end{equation*}$

We can also find $sin(A+B)$

Remember, $sin\theta=cos(\frac{\pi}{2}-\theta)$

$\begin{equation*}sin(A+B)=cos(\frac{\pi}{2}-(A+B))\end{equation}$

$\begin{equation*}sin(A+B)=cos((\frac{\pi}{2}-A)-B)\end{equation}$

$\begin{equation*}sin(A+B)=cos(\frac{\pi}{2}-A)cosB+sin(\frac{\pi}{2}-A)sinB\end{equation}$

(3) $\begin{equation*}sin(A+B)=sinAcosB+cosAsinB\end{equation*}$

We can use equation $2$ to find $sin(A-B)$

$\begin{equation*}sin(A-B)=sin(A+(-B))\end{equation}$

$\begin{equation*}sin(A+(-B))=sinAcos(-B)+cosAsin(-B)\end{equation}$

$\begin{equation*}sin(A+(-B))=sinAcos(B)-cosAsin(B)\end{equation}$

(4) $\begin{equation*}sin(A-B)=sinAcos(B)-cosAsin(B)\end{equation*}$

And we can use both the sine and cosine identities to find $tan(A+B)$

Remember $tan\theta=\frac{sin\theta}{cos\theta}$

$\begin{equation*}tan(A+B)=\frac{sin(A+B)}{cos(A+B)}\end{equation}$

$\begin{equation*}=\frac{sinAcosB+cosAsinB}{cosAcosB-sinAsinB}\end{equation}$

$\begin{equation*}=\frac{sinAcosB+cosAsinB}{cosAcosB-sinAsinB}\times \frac{cosAcosB}{cosAcosB}\end{equation}$

$\begin{equation*}=\frac{\frac{sinAcosB}{cosAcosB}+\frac{cosAsinB}{cosAcosB}}{\frac{cosAcosB}{cosAcosB}+\frac{sinAsinB}{cosAcosB}}\end{equation}$

$\begin{equation*}=\frac{tanA+tanB}{1-tanAtanB}\end{equation}$

(5) $\begin{equation*}tan(A+B)=\frac{tanA+tanB}{1-tanAtanB}\end{equation*}$

and

(6) $\begin{equation*}tan(A-B)=\frac{tanA-tanB}{1+tanAtanB}\end{equation*}$

$\begin{equation*}cos(A\pm B)=cosAcosB\mp sinAsinB\end{equation}$

$\begin{equation*}sin(A\pm B)=sinAcosB\pm cosAsinB\end{equation}$

$\begin{equation*}tan(A \pm B)=\frac{tan A \pm tanB}{1 \mp tanAtanB}\end{equation}$

Leave a Comment

Filed under Addition and Subtraction Identities, Identities, Non-Right Trigonometry, Simplifying fractions, Trigonometry, Year 11 Mathematical Methods, Year 11 Specialist Mathematics

Tagged as addition and subtraction trig identities, trig identities

April 21, 2025 · 11:08 am

Area/Geometry Problem

This problem is from The Geometry Forum Problem of the Week June 1996

In triangle ABC, AC=18 and D is the point on AC for which AD=5. Perpendiculars drawn from D to AB and CB have lengths of 4 and 5 respectively. What is the area of triangle ABC?

I put together a diagram (in Geogebra)

Add points P and Q

Triangle APD and triangle DQC are right angled. Using pythagoras, $AP=3$ and $QC=12$

$BQDP$ is a cyclic quadrilateral and $BD$ is the diameter. I am not sure if this is useful, but it is good to notice.

$\begin{equation*}sin(A+B+C)=sin(180)=0\end{equation}$

$\begin{equation*}sin((A+C)+B)=sin(A+C)cosB+sinBcos(A+C)=0\end{equation}$

$\begin{equation*}cosB(sinAcosC+sinCcosA)+sinB(cosAcosC-sinAsinC)=0\end{equation}$

$\begin{equation*}cosB(\frac{4}{5}\times\frac{12}{13}+\frac{5}{13}\times\frac{3}{5})+sinB(\frac{3}{5}\times\frac{12}{13}-\frac{4}{5}\times\frac{5}{13})=0\end{equation}$

$\begin{equation*}cosB(\frac{48}{65}+\frac{15}{65})+sinB(\frac{36}{65}-\frac{20}{65})=0\end{equation}$

$\begin{equation*}\frac{63}{65}cosB+\frac{16}{65}sinB=0\end{equation}$

$\begin{equation*}63cosB+16sinB=0\end{equation}$

$\begin{equation*}63+16tanB=0\end{equation}$

$\begin{equation*}tanB=\frac{-63}{16}\end{equation}$

If $tanB=\frac{-63}{16}$ then $sinB=\frac{63}{65}$

Now,

$\begin{equation*}\frac{y+12}{sinA}=\frac{18}{sinB}\end{equation}$

$\begin{equation*}y+12=\frac{4}{5}(18)\frac{65}{63}\end{equation}$

$\begin{equation*}y+12=\frac{104}{7}\end{equation}$

Hence the Area is

$\begin{equation*}A=\frac{1}{2}(18)(\frac{104}{7})sinC\end{equation}$

$\begin{equation*}A=\frac{1}{2}(18)(\frac{104}{7})\frac{5}{13}\end{equation}$

$\begin{equation*}A=\frac{360}{7}=51.43\end{equation}$

Leave a Comment

Filed under Area, Finding an area, Geometry, Identities, Non-Right Trigonometry, Pythagoras, Trigonometry

Tagged as area, area of triangles, problem solving

February 4, 2025 · 9:17 am

Differentiating the Tangent Function

Remember $tan(x)=\frac{sin(x)}{cos(x)}$ .

I use the quotient rule to differentiate $f(x)=tan(x)$ .

(1) $\begin{equation*}\frac{d}{dx}(\frac{f(x)}{g(x)})=\frac{f'(x)g(x)-g'(x)f(x)}{[g(x)]^2}\end{equation*}$

If $h(x)=tan(x)=\frac{sin(x)}{cos(x)}$ then from equation $1$

(2) $\begin{equation*}h'(x)=\frac{cos(x)cos(x)-(-sin(x)sin(x))}{[cos(x)]^2}\end{equation*}$

(3) $\begin{equation*}h'(x)=\frac{cos^2(x)+sin^2(x)}{cos^2(x)}\end{equation*}$

Remember the Pythagorean identity

(4) $\begin{equation*}sin^2(x)+cos^2(x)=1\end{equation*}$

Hence

$\begin{equation*}h'(x)=\frac{1}{cos^2(x)}=sec^2(x)\end{equation}$

(5) $\begin{equation*}\frac{d}{dx}tan(x)=sec^2(x)\end{equation*}$

Leave a Comment

Filed under Calculus, Differentiation, Differentiation, Identities, Quotient Rule, Trigonometry, Year 12 Mathematical Methods

Tagged as differentiating tan, differentiating trig

February 3, 2025 · 9:11 am

Differentiating Trigonometric Functions

In the last post we looked at two trig limits:

(1) $\begin{equation*}\lim_{x \to 0}\frac{sin(x)}{x}=1\end{equation*}$

(2) $\begin{equation*}\lim_{x \to 0}\frac{1-cos(x)}{x}=0\end{equation*}$

We are going to use these two limits to differentiate sine and cosine functions from first principals.

$\begin{equation*}f(x)=sin(x)\end{equation}$

$\begin{equation*}f'(x)=\lim\limits_{h \to 0}\frac{sin(x+h)-sin(x)}{h}\end{equation}$

Use the trig identity

$\begin{equation*}sin(A+B)=sinAcosB+sinBcosA\end{equation}$

$\begin{equation*}f'(x)=\lim\limits_{h \to 0}\frac{sin(x)cos(h)+sin(h)cos(x)-sin(x)}{h}\end{equation}$

$\begin{equation*}f'(x)=\lim\limits_{h \to 0}(\frac{sin(x)(cos(h)-1)}{h}+\frac{sin(h)cos(x)}{h})\end{equation}$

$\begin{equation*}f'(x)=sin(x)\lim\limits_{h \to 0}(\frac{(cos(h)-1)}{h}+cos(x)\lim\limits_{h \to 0}\frac{sin(h)}{h}\end{equation}$

$\begin{equation*}f'(x)=sin(x)\lim\limits_{h \to 0}(\frac{-(-cos(h)+1)}{h}+cos(x)\lim\limits_{h \to 0}\frac{sin(h)}{h}\end{equation}$

Evaluate the limits

$\begin{equation*}f'(x)=sin(x)\times 0+cos(x)\times (1)=cos(x)\end{equation}$

Hence, $\frac{d}{dx}sin(x)=cos(x)$ .

Now we are going to do the same for $f(x)=cos(x)$ .

$\begin{equation*}f'(x)=\lim\limits_{h \to 0}\frac{cos(x+h)-cos(x)}{h}\end{equation}$

Use the trigonometric identity

$\begin{equation*}cos(A+B)=cosAcosB-sinAsinB\end{equation}$

$\begin{equation*}f'(x)=\lim\limits_{h \to 0}\frac{cos(x)cos(h)-sin(x)sin(h)-cos(x)}{h}\end{equation}$

$\begin{equation*}f'(x)=\lim\limits_{h \to 0}\frac{cos(x)(cos(h)-1)-sin(x)sin(h)}{h}\end{equation}$

$\begin{equation*}f'(x)=cos(x)\lim\limits_{h \to 0}\frac{-(1-cos(h))}{h}-sin(x)\lim\limits_{h \to 0}\frac{sin(h)}{h}\end{equation}$

Evaluate the limits

$\begin{equation*}f'(x)=cos(x)\times(0)-sin(x)\times (1)=-sin(x)\end{equation}$

Hence $\frac{d}{dx} cos(x)=-sin(x)$

1 Comment

Filed under Calculus, Differentiation, Identities, Trigonometry, Year 12 Mathematical Methods

Tagged as differentiation, trig differentiation, trig identities, trig limits, trigonometric differentiation, trigonometric identities, trigonometric limits

Category Archives: Identities

Trigonometric Equation

Trigonometric Exact Values

Matrices -Linear Transformations (Rotation)

Example

Trig Identities and Exact Values

Trigonometric Identities – Product to Sum

Examples

Trigonometric Exact Value

Trig Identities – Addition and Subtraction

Area/Geometry Problem

Differentiating the Tangent Function

Differentiating Trigonometric Functions

Recent Posts

Categories

Archives