Napoleon’s Theorem

This is one of those theorems, where the name is probably not the person who discovered it. Check out the Wikipedia entry for more information on the history.

Napoleon’s theorem states that if equilateral triangles are constructed on the sides of any triangle, either all outward or all inward, the lines connecting the centres of those equilateral triangles themselves form an equilateral triangle.

I am just going to look at the outward triangles.

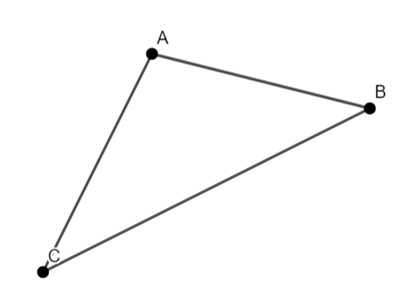
Construct equilateral triangles on the edges AB, BC and AC.

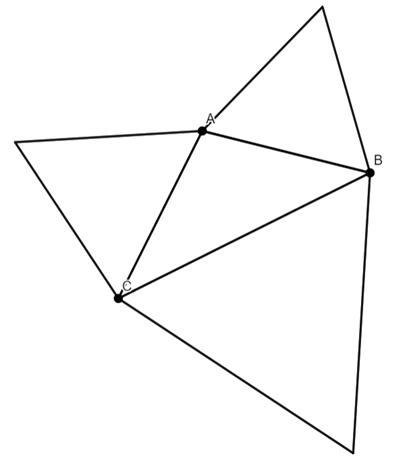
Find the centre (Centroid) of these triangles.

Note: The centroid is the point of intersections of the medians.

A median is the line from a vertex to the midpoint of the opposite side.

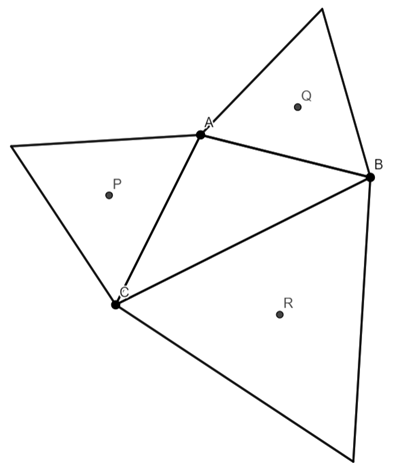
We are going to prove that triangle PQR is equilateral

I am going to add AP, AQ, QB, BR, RC and CP

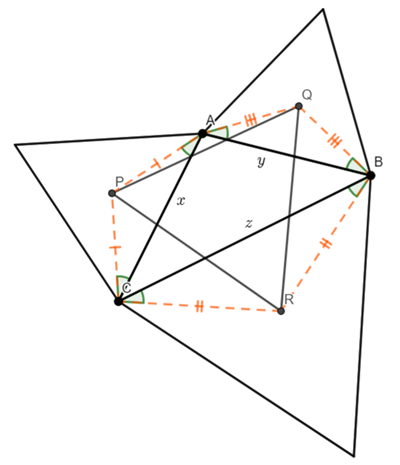
As P, Q and R are the centroids, PA=PC, QA=QB, and RB=RC.

Therefore triangles AQB, BRC, and CPA are isosceles.

The angles QAB, QBA, RBC, RCB, PCA, PAC (the shaded angles in the above diagram) are 30 degrees, because the line bisects the angle (and the angle is 60 degrees because the triangles are equilateral).

Now for the maths.

$\textnormal {Let}\ AB=y,\ AC=x,\ BC=z$

$\textnormal {Let}\ \angle{CAB}=\theta,\ \angle{ABC}=\alpha,\ \angle{BCA}=\gamma$

$\therefore\angle{PAQ}=(60+\theta),\ \angle{QBR}=(60+\alpha),$

$\angle{RCP}=(60+\gamma)$

PA is 2/3 the length of the median.

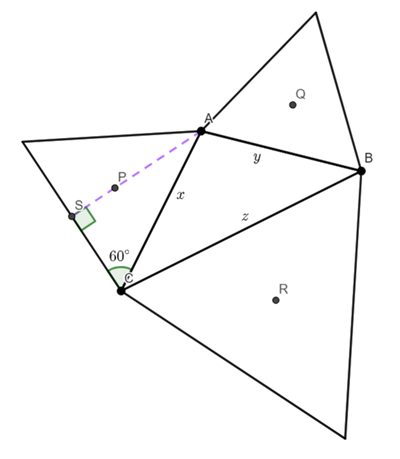
AS is the median

$sin(60)=\frac{AS}{x}$

$AS=xsin(60)$

$AS=\frac{\sqrt{3}}{2}x$

Hence,

$PA=\frac{2}{3}\frac{\sqrt3}{2}x$

$PA=\frac{\sqrt{3}}{3}x$

$PA=\frac{x}{\sqrt{3}}$

In the same manner,

$QA=\frac{y}{\sqrt{3}}\ \textnormal {and} \ BR=\frac{z}{\sqrt{3}}$

Using the cosine rule

$(PQ)^2=(\frac{x}{\sqrt{3}})^2+(\frac{y}{\sqrt{3}})^2-2\frac{x}{\sqrt{3}}\frac{y}{\sqrt{3}}cos(60+\theta)$

$(PQ)^2=\frac{x^2}{3}+\frac{y^2}{3}-\frac{2xy}{3}cos(60+\theta)$

$\textnormal{Use a trigonometric identity to expand}\ cos(60+\theta)$

$(PQ)^2=\frac{x^2}{3}+\frac{y^2}{3}-\frac{2xy}{3}(cos60cos\theta-sin60sin\theta)$

$(PQ)^2=\frac{x^2}{3}+\frac{y^2}{3}-\frac{2xy}{3}(\frac{1}{2}cos\theta-\frac{\sqrt{3}}{2}sin\theta)$

Now from the original triangle ABC

$cos\theta=\frac{x^2+y^2-z^2}{2xy}\ \textnormal {and}\ A=\frac{1}{2}xysin\theta\ \textnormal {where} A\ \textnormal {is the area.}$

$\textnormal{Substitute into}\ (PQ)^2$

$(PQ)^2=\frac{x^2}{3}+\frac{y^2}{3}-\frac{2xy}{3}(\frac{x^2+y^2-z^2}{4xy}-\frac{\sqrt{3}A}{xy})$

Multiply both sides by 3 and expand and simplify

$3(PQ)^2=x^2+y^2-2xy(\frac{x^2+y^2-z^2}{4xy}-\frac{\sqrt{3}A}{xy})$

$3(PQ)^2=x^2+y^2-(\frac{x^2}{2}+\frac{y^2}{2}-\frac{z^2}{2}-2\sqrt{3}A)$

$3(PQ)^2=\frac{x^2}{2}+\frac{y^2}{2}+\frac{z^2}{2}+2\sqrt{3}A$

We are going to do the same for QR

$(QR)^2=\frac{y^2}{3}+\frac{z^2}{3}-\frac{2yz}{3}(cos(60+\alpha)$

$(QR)^2=\frac{y^2}{3}+\frac{z^2}{3}-\frac{2xy}{3}(cos60cos\alpha-sin60sin\alpha)$

$(QR)^2=\frac{y^2}{3}+\frac{z^2}{3}-\frac{2xy}{3}(\frac{1}{2}cos\alpha-\frac{\sqrt{3}}{2}sin\alpha)$

Once again, from triangle ABC

$cos\alpha=\frac{y^2+z^2-x^2}{2yz}\ \textnormal {and}\ A=\frac{1}{2}zysin\alpha\ \textnormal {where} A\ \textnormal {is the area.}$

$\textnormal{Substitute into}\ (QR)^2$

$(QR)^2=\frac{y^2}{3}+\frac{z^2}{3}-\frac{2yz}{3}(\frac{y^2+z^2-x^2}{4yz}-\frac{\sqrt{3}A}{yz})$

Multiply both sides by three and expand and simplify

$3(QR)^2=y^2+z^2-2yz(\frac{y^2+z^2-x^2}{4yz}-\frac{\sqrt{3}A}{yz})$

$3(QR)^2=y^2+z^2-(\frac{y^2}{2}+\frac{z^2}{2}-\frac{x^2}{2}-2\sqrt{3}A)$

$3(QR)^2=\frac{x^2}{2}+\frac{y^2}{2}+\frac{z^2}{2}+2\sqrt{3}A$

We can do the same for PR

$(PR)^2=\frac{x^2}{3}+\frac{z^2}{3}-\frac{2xz}{3}cos(60+\gamma)$

$(PR)^2=\frac{x^2}{3}+\frac{z^2}{3}-\frac{2xz}{3}(cos60cos\gamma-sin60sin\gamma)$

$(PR)^2=\frac{x^2}{3}+\frac{z^2}{3}-\frac{2xz}{3}(\frac{1}{2}cos\gamma-\frac{\sqrt{3}}{2}sin\gamma)$

And from triangle ABC

$cos\gamma=\frac{x^2+z^2-y^2}{2xz}\ \textnormal{and}\ A=\frac{1}{2}sin\gamma\ \textnormal{where A is the area.}$

$\textnormal{substitute into}\ (PR)^2$

$(PR)^2=\frac{x^2}{3}+\frac{y^2}{3}-\frac{2xz}{3}(\frac{x^2+z^2-y^2}{4xz}-\frac{\sqrt{3}A}{xz})$

Multiply both sides by three and expand and simplify

$3(PR)^2=\frac{x^2}{2}+\frac{y^2}{2}+\frac{z^2}{2}+2\sqrt{3}A$

Hence, PQ=PR=RQ and triangle PQR is equilateral.

Leave a Reply Cancel reply

Recent Posts

Categories

Archives