September 24, 2024 · 3:30 am
↓ Jump to Comments

Optimisation

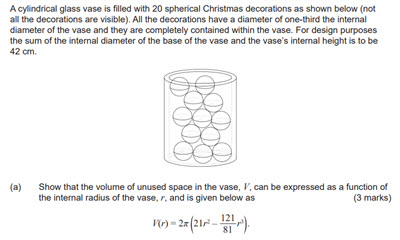
An optimisation question from the 2019 ATAR Mathematics Methods exam.

I always like optimisation questions. There is a nice process to follow:

  • Find the function to optimise (in terms of one variable).
  • Find the stationary points.
  • Find the nature of the stationary points.
  • Find the maximum or minimum.
(a) Volume of the cylinder V=\pi r^2h
42=2r+h
h=42-2r
\therefore V_C=\pi r^2(42-2r)
Volume of spherical decorations V_S=\frac{4}{3}\pi( r_s)^3 where r_s=\frac{r}{3}
V_S=\frac{4\pi r^3}{81}
Volume unused space V=\pi r^2(42-2r)-20(\frac{4\pi r^3}{81})
V=2\pi (21r^2-r^3-\frac{40r^3}{81})
V=2\pi (21r^2-\frac{81r^3}{81}-\frac{40r^3}{81})
V=2\pi (21r^2-\frac{121r^3}{81})

(b) V=2\pi (21r^2-\frac{121r^3}{81})
\frac{dV}{dr}=2\pi (42r-\frac{121r^2}{27})
\frac{dV}{dr}=0
0=42r-\frac{121r^2}{27}
0=r(42-\frac{121r}{27})
r=0 or r=\frac{1134}{121}=9.372

\frac{d^2V}{dr^2}=2\pi (42-\frac{242r}{27})
(\frac{d^2V}{dr^2})_{|r=9.372}=-42
\therefore r=9.372 is a maximum.

Dimensions of the vase, internal diameter=18.7cm internal height=23.3cm

(c) Maximum volume of empty space =2\pi (21r^2-\frac{121r^3}{81})=3863.08cm^3
Volume of one sphere =\frac{4}{3}\pi r^3=3448.03cm^3

There is enough unused space for one extra decoration, but it would depend on how they are packed.

Leave a Comment

Filed under Differentiation, Optimisation, Year 12 Mathematical Methods

Tagged as , ,

Leave a Reply

Your email address will not be published. Required fields are marked *